Back of the Envelope

A  useful skill not often taught is doing back of the envelope calculations,¹ so called because they can be, sometimes are, done on the back of an envelope destined for the waste basket. The idea is to use approximate data and approximate models to try to get the right answer to a question to within about a factor of ten in either direction. One of my earlier posts was an example. Here are some more.

How long cars last: It’s not uncommon to hear complaints about how quickly cars, especially American cars, wear out, sometimes linked to the claim that they are designed to wear out so you will have to buy a new one. Along similar lines, American consumers are sometimes pictured as routinely buying a new car every two years.

It is easy enough to look up how many cars are on the road and how many are produced each year. The first time I did the calculation I used the statistical Abstract, this time I used Google. As of September 2022 there were 290.8 million cars in the U.S. In 2022, the auto industry in the United States sold approximately 13.75 million light vehicle units. Dividing the first number by the second tells us that the average car lasts about 21 years.²

Population density: One occasionally sees concerns that, as population grows, housing swallows up land needed for farming. To see how plausible they are I start by estimating the amount of land in the US occupied by housing. A large house nowadays has 3000 square feet of floor area, typically on two floors, and is occupied by four people, which means 1500/4 square feet of land per person. That’s surely a high figure, since most people don’t live in houses that large and a considerable part of the population is in urban areas in housing with more than two stories. Round numbers make calculation easier, so call it 200 square feet per person. An acre is 200'x200', so that gives you 200 people per acre. There are 640 acres in a square mile, so (rounding down for simplicity) about 100,000 people per square mile. The U.S. population is about 300 million, so the total area occupied by housing should be about 3000 square miles.

The U.S. is, very roughly, 3000 miles east to west and 1000 miles north to south. So housing occupies about 1/1000th of its area.

This is a very approximate figure, produced without looking up anything. And it only includes housing, not lawns, streets, grocery stores, and the like. But it is still enough to show that mental images of people packed in like sardines due to too many people in not enough space, along with the associated arguments about how rats behave when there are too many of them in a cage, have to be wrong. There are areas where human construction occupies a large fraction of the land area. But the reason — at least in the U.S. — is not that there are too many people for too little land but that many people choose to live in densely populated areas.

One reason people overestimate population density is that they form their opinion based on what they see around them — and people spend most of their time in places where there are people. They are averaging population density over people rather than over acres, asking how densely populated an area the average person lives in rather than how densely populated the average acre is.

One way of correcting that error is to observe a sample that is not biased in that way by, for instance, looking down from an airplane window when flying across the country. What you see is much more likely to be corn fields, mountains, desert or forest than a sea of rooftops.

Asteroid Strikes: The risk of asteroid strikes has two relevant dimensions: How much damage would an asteroid of a given size do and how likely is an asteroid of that size to hit the earth.

Start with a case we have good evidence on, the Tunguska event. In 1908 something caused a very large explosion over Siberia, roughly equivalent to a hydrogen bomb; the current preferred theory seems to be that it was an airburst of a large meteor or comet fragment. It knocked down trees over an area of about 2000 square kilometers.

Dropping a hydrogen bomb from time to time at some random location sounds pretty scary; perhaps we were just extraordinarily lucky that it hit Siberia instead of Manhattan. A simple back of the envelope calculation can tell us about how lucky we were.

The earth is a globe with a radius of about 4000 miles, roughly 7000 kilometers. The area of a sphere is 4π times the radius squared, making the surface area of the earth about six hundred million square kilometers, so the area over which trees were knocked down by the Tunguska explosion represents about 1/300,000 of the area of the earth. The current population of the earth is between six and seven billion. If we assume that the area over which trees were knocked down is about the same as the area over which humans would be killed, the average death toll from a Tunguska event would be about 20,000. That is a lot of people but hardly a global catastrophe—about half the number killed in the US each year in auto accidents, about one three hundredth of the number killed in the Holocaust.

How likely is a Tunguska event? It is unlikely that one could have occurred in the past century without being observed, given the seismographic effect, which registered as far off as Washington D.C. How much farther back one can push that argument I do not know, so I will assume that such events happen at a rate of one a century. If so, the average mortality from such events is about 200 deaths/year. Every death matters, but there are a lot of problems in the world that do a great deal more damage than that.

There is a good deal left out of these calculations. For one thing I don’t know how the area of damage from a sea strike would compare with that from a land strike or how easily it would have been observed if it happened during the past century, and a sea strike is considerably more likely than a land strike. But they are enough to give us a rough scale for the problem.

So far I have considered only things on the scale of the Tunguska event but we know that there have been, at very long intervals, much larger meteor strikes. One famous one about sixty million years back is sometimes referred to as the Dinosaur Killer, on the theory that its effects killed off the dinosaurs. My geologist wife objects to that label on the grounds that lots of other things went extinct at the same time; the technical term is the K-T event. The evidence for several earlier large strikes with less drastic consequences is preserved as astroblemes, geological structures believed to be the result of asteroids hitting the earth. So let’s guess that they occur at a rate of one every sixty million years. We don’t know how many people would be killed by a strike on that scale but the upper limit is everyone, so use that for a very rough calculation. Dividing about eight billion people by about sixty million years gives us a mortality rate of about a hundred and thirty-three people a year.

Here again, my calculations leave a lot out. Mass extinctions on the scale of the K-T event occur at a rate considerably below one every sixty million years; there are fewer of them than there are astroblemes. That suggests that perhaps I should have divided by 300 million or so instead of sixty million. On the other hand, I have not considered events intermediate between the two categories, infrequent enough to be left out of the historical record and small enough to be left out of the fossil record but still bigger than Tunguska and more frequent than K-T. But I think my calculations are sufficient to show that annual mortality due to asteroid strikes is tiny compared to other sources.

One final question is whether annual mortality is all that matters. Perhaps we ought to consider not only individual lives but the survival of our species and our civilization. Seen from that standpoint, if an asteroid strike really does kill everyone the cost, as evaluated by those presently living, might be considerably larger than the number of lives lost. My own guess is that even something on the scale of the K-T event would not wipe out either our species or our civilization but I might be wrong.

Drinking Water: During a recent drought there were signs on the tables of California restaurants explaining that, because of the water shortage, they only bring drinking water if you ask for it. The obvious question is whether the amounts involved are large enough to matter. It is straightforward to estimate how much water is being saved per person per year. You can go from that either to an estimate of the size of the local reservoir and the number of people it serves or the amount of water used for some other purpose, such as watering lawns or flushing toilets, or—with a little searching—to per capita water consumption in the U.S., and compare the numbers.

Nutrition, Obesity, Cost

In one online exchange, a poster commented on how extraordinary it was that poor people in our society are fatter than not-poor people. Someone responded that the reason was that more nutritious food was more expensive. She did not go into details but my guess is that she was thinking of fast food as cheap; I have seen other people make the argument in that form.

I responded by offering home made bread and lentils as examples of inexpensive but nutritious foods. Another poster responded with the claim that home made bread, while tasty and nutritious, was more expensive than "the cheap and nasty supermarket bread." So I did some price comparisons, getting my price and nutrition information off the web.

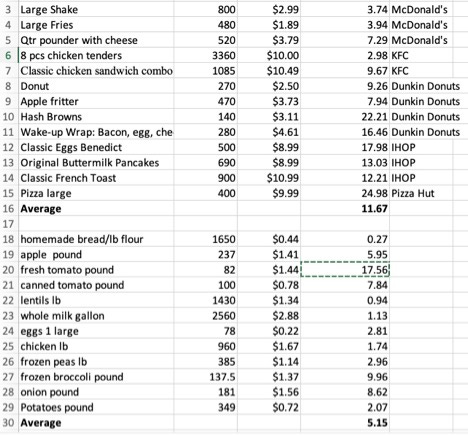
Flour, the main ingredient in home made bread, costs about $.44/lb at Walmart and has about 1650 calories/lb, so flour for homemade bread, or anything else made mostly of flour, costs about $.27/1000 calories. Wonder Bread, the classic example of supermarket bread, has 1400 calories/loaf and costs $2.92/loaf at Walmart. So about $2.09/1000 calories, almost eight times as much.

That does not include any cost for the time making the bread, which is appropriate if the cook has nothing else to do with his time or regards bread making as recreation. If not, figure about a quarter hour of working time per pound of flour and price it at $10/hour, since we are talking about poor people, and that raises the cost of home made bread to about $2.77/1000 calories, still a little less than Wonder Bread.

Comparing lentils to fast food, 1 pound of lentils has about 1430 calories and costs $1.34 at Walmart, so about $.94/1000 calories. Time spent cooking them, if you are not doing anything fancy, is negligible. A McDonalds Quarter Pounder with cheese has 520 calories and costs $3.79,  so about $7.29/1000 calories.

That answers the particular question that came up in the online argument. To get a more general answer I worked out the price of a thousand calories for a selection of fast foods and a selection of cooking ingredients;³ my calculations are shown below. The fast foods varied from $2.98 for KFC chicken tenders to 24.98 for a large pizza, with an average cost of $11.67/1000 calories. The cooking ingredients varied from 17.56 (fresh tomatoes, but canned cost only $7.84) to $.27 for flour. The average was $5.15/1000 calories; the fresh tomatoes were the only item for which the cost was more than ten dollars.

This is only a back of the envelope calculation with an arbitrary selection of items so my averages should not be taken very seriously but it suggests that eating fast food is a good deal more expensive in money than cooking for yourself.

I am not making any allowance for cooking time. Readers who are cooks may want to work out for themselves the time cost of the fastest nutritious dishes they can think of, remembering that most Americans, even most poor Americans, have refrigerators with freezing compartments and that making a big pot of chili or beef stew, freezing most if it in meal sized portions and microwaving as needed takes a lot less time than cooking each meal separately.

1. Sometimes referred to as Fermi Estimates, possibly based on a story that Enrico Fermi roughly calculated the yield of the first atomic bomb test by tossing a handful of paper shards into the air when he saw the flash and measuring how far they were transported by the shock wave.

A commenter on my blog pointed at a textbook draft and course materials from a Cal Tech course on the subject, another at a published book on it.

2. The figure is for cars and light trucks but the latter category includes SUV’s, minivans and pickups, all of which count for the “vehicles on the road” number. As best I can tell it is for vehicles sold in the US not vehicles produced so I don’t need to adjust it to take account of net imports.

3. Most of the cooking ingredient figures are from the Walmart web page which gives prices and, in most cases, calories.